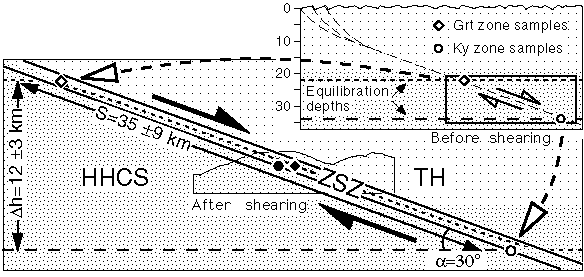
7.2 Displacement along the ZSZTo estimate the amount of ductile shearing that was accommodated by the ZSZ, we resorted to a simple trigonometric model which is presented in fig. 7.2.1. This model is based on the observation that, along a horizontal profile across the ZSZ, one can see the successive apparition of increasingly metamorphosed rocks, which equilibrated at very different depths, but are now at the same structural level. The net slip (s) required to bring two such rocks, initially separated by a vertical distance (DH), to the same structural level within a shear zone (dipping at an angle a) can easily be calculated by:
s = DH / sina. (1)
The equilibration depth of the various metamorphic zones is given either by thermobarometric results or by petrographic evidences and the present dip of the shear zone is known (20°). It is thus possible to calculate the net slip along the ZSZ.
|
|
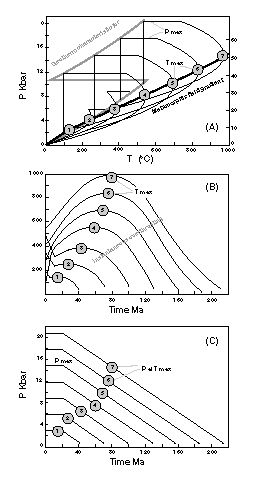
Fig. 7.2: P-T paths (A), T-time paths (B) and P-time paths (C) showing the theoretical evolution of 7 rocks after instantaneous overthrusting (Spear, 1993). Rocks 1, 2 and 3 are from the overthrusting unit and 4, 5, 6 and 7 are from the overthrusted unit. The circles labelling each rock path have been placed on the peak metamorphic conditions definrd by T max. (A), The line drawn through the loci of thermal maxima corresponds to the "metamorphic field gradient". (B) Note that there is a progression in the time of Tmax; the deeper the rock, the later it reaches T max. (C) Note that the value of P at Tmax is lower than the maximum value of pressure (Pmax) for each rock. |
|
Several considerations indicate that this value of 35 ± 9 km is a minimum value for the displacement along the ZSZ: - The kyanite zone to garnet zone rocks represent only part of the sheared sequence (fig. 5.8). The deformation accommodated in the biotite zone has not been considered as no quantitative pressure constraints can be obtained for these assemblages. Additionally, more brittle extension in higher levels is also not quantified. It is however very likely that most of the deformation was concentrated in the high-grade, ductile part of the ZSZ and that the lower-grade rocks accommodated less shearing (e.g. Hacker and Mosenfelder, 1996). Petrographic evidences (staurolite out) indicate that the lowermost part of the kyanite zone equilibrated at higher pressures than those indicated by the thermobarometrical results. On the basis of these arguments, even a cautious estimation of the vertical distance between the equilibration depth of biotite zone and the lowermost kyanite zone results in a value of DH ~16 km. With this value, the net slip of the ZSZ would be s ~ 47 km. - The pressure estimate for peak conditions in the Migmatite Zone (fig. 5.21) indicates an equilibration depth of 43 ± 4 km (Table 5.1). Although this estimate is calculated with a different methodology compared to the ZSZ samples, it fits in with the metamorphic field gradient defined by these latter rocks, as well as with another estimate for comparable migmatites (fig. 5.21, sample MPL). The difference in equilibration depths between the Migmatite Zone and the ZSZ garnet zone rocks implies a vertical displacement DH 21 ± 4 km and a net slip s 61 ± 12 km to bring these samples to the same horizontal level. - Thirdly, estimates for the ZSZ net slip are highly sensitive to the dip of the structure. Because of the late large-scale doming of the HHCS in Zanskar, the original dip of the ZSZ was possibly less than its present dip of 20°. In the Qomolangma (Mt. Everest) region, where no doming is observed, the dip of the STDS varies between 15° to 5° (Burchfiel et al., 1992). Recent seismic data suggest that the dip of the STDS in the eastern Himalaya is approximately 12°, before flattening out at 20 km depth (Makovsky et al., 1996; Alsdorf et al., 1998; Hauck et al., 1998). For an original dip of 12° and a vertical displacement DH~ 21 ± 4 km, the net slip of the ZSZ could thus have been up to s ~100 ± 20 km. |
The net slip along the ZSZ in central Zanskar was estimated by Herren (1987) to be at least 25 km, on the basis of a geometrical model for the shearing of isograds. This model allows to calculate the net slip (s) along the shear zone using the equation:
s = (b / sinb)(h / h' - cosb) (2)
where h is the thickness of a supposedly unsheared rock sequence between two known isograds outside the ZSZ (measured in the western Suru area), h' is the thickness of the same sequence sheared within the ZSZ, b is the width of the ZSZ, and b is the dip of the ZSZ relative to the dip of the isograds outside the shear zone (b = 20°).
Equation (2) can be developed as:
s = (b*h / h'*sinb) - (b*cosa / sinb) (2.1)
s = (h*(b/h') / sinb) - (b / tanb) (2.2)
(2.2) is very similar to our own equation (1) because h*(b/h') is equivalent to DH and the term (b / tanb) only implies that one considers sampling perpendicular to the shear zone boundaries, instead of along a horizontal profile. The difference is anyway negligible. The major difference between the two equations is that Herren considers the angle b between the dip of the ZSZ and the dip of the isograds, where we only consider the dip of the shear zone (a)
Although this approach is broadly comparable to ours, it is based on some poorly constrained parameters. First, Herren (1987) measured a thickness h 6.3 km between the garnet to sillimanite isograds outside the ZSZ. This value appears surprisingly small compared to the DH = 12 ± 3 km of initial vertical distance between the garnet zone and kyanite zone samples, as indicated by our thermobarometry results. This difference suggests that, even outside the ZSZ, the isograds may have been thinned by the late- to post-metamorphic extensional structures scattered throughout the HHCS of Zanskar (Kündig, 1989). This process would lead to an underestimation of the original thickness of the sequence bound by known isograds, and therefore of the displacement along the ZSZ, using Herren's approach. In contrast, our estimate of the vertical displacement based on P-T results for peak conditions would not be affected. Secondly, Herren (1987) calculated the total thickness of the sequence before shearing as h ( b / h'). Because b and h' are both comparably small (0.35 and 0.25 km respectively in Herren, 1987), even small uncertainties in these two values have a major influence on the estimation of the total original thickness of the sequence now sheared within the ZSZ, and thus on the total movement. Thirdly, Herren's calculation of the net slip is dependant on the angle between the shear zone and the isograds which is difficult to estimate precisely.
Using the thermobarometric and the petrographic information to estimate the original thickness of the sheared metamorphic sequence proves to be much more accurate, as it allows to avoid most of the weakly constrained parameters used in Herren's purely geometrical model. Moreover our final calculation of the net slip along the ZSZ is independent of the original angle the isograds formed with the extensional structure.
| Introduction | Timing of extensional shearing |
©Pierre Dèzes